Fundamentals of Statistics contains material of various lectures and courses of H. Lohninger on statistics, data analysis and chemometrics......click here for more.

Home Univariate Data Distributions Common Distributons Continuous Distributions Pareto-Verteilung
See also: Cauchy Distribution 

Pareto Distribution

Definition
Normally, the parameter K is known and represents the lower border of the data. The parameter λ has to be adjusted to the data to obtain a best fit.

The Pareto distribution has been described for the first time by V. Pareto, an Italian economist, who discovered that a small portion of the population owns a large portion of the gross national capital (80-20-rule: 20% of the population own 80% of the capital).
Graphic Representation  
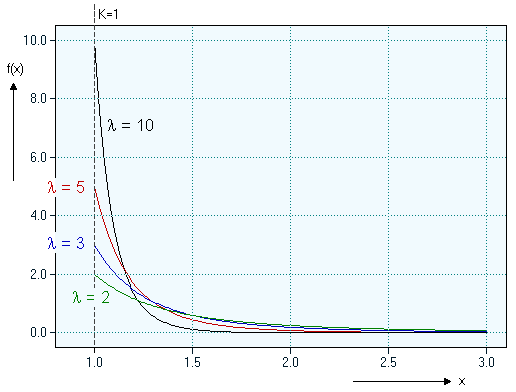
The diagram at the left shows the Pareto distribution with the parameters K=1 and λ=2, 3, 5 and 10
Applications A few examples of data following a Pareto distribution:
  • the size of meteorites (many small, few large ones)
  • the size of settlements (few mega cities, many small villages)
  • the wealth of persons
  • the size of sand grains

Mean μ = Kλ/(λ-1); λ > 1
Variance σ = K2λ/(λ-1)2/(λ-2); λ > 2

Home Univariate Data Distributions Common Distributons Continuous Distributions Pareto-Verteilung