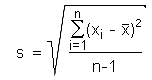Fundamentals of Statistics contains material of various lectures and courses of H. Lohninger on statistics, data analysis and chemometrics......click here for more.

Home Univariate Data Measures of Variation Standard Deviation
See also: variance, precision of results, mean, quartile, coefficient of variation, Chebyshev's Theorem 

Standard Deviation

The standard deviation is the positive square root of the variance, and is depicted by s for samples, or by σ for populations. The standard deviation is a useful measure of variability because of its mathematical tractability:

There is often some confusion about the standard deviation and its interpretation. One should carefully distinguish between the formal definition of the standard deviation and the interpretation of it. The standard deviation as a numerical value can always be calculated provided that there are enough samples available. In contrast to this, the interpretation of the standard deviation as a measure of spread can be fully utilized only if the type of the distribution is known. However, the theorem of Chebyshev gives some guidelines for any (!) distribution.

In the case of a normal distribution the following rules of thumb can be applied:

(μ σ) contains about 70% of the observations
(μ 2σ) contains about 95% of the observations
(μ 3σ) contains more than 99% of the observations
Home Univariate Data Measures of Variation Standard Deviation