Mean

The mean is commonly called the average. From a mathematical standpoint there are several types of means which are legitimate in various scenarios:

Arithmetic Mean	It is calculated by adding all the values and dividing the sum by the number of values. Let xi represent the values of a variable X, with i = 1, 2, ..., n. The mean  is then defined as: Note that when the word "mean" is used without a modifier, it usually refers to the arithmetic mean as defined by the formula above.
Harmonic Mean	In the case of calculations where reciprocal values are important (e.g. involving proportions, or velocities at constant distances1) the calculation of the mean should be based on the harmonic mean, which is defined by the reciprocal of the mean of the reciprocals. The harmonic mean never becomes greater than the arithmetic mean.
Geometric Mean	The geometric mean has to be used for averaging multiplicative factors (e.g. the average increase of stock prices). It is calculated as the n-th root of the product of all factors: The geometric mean is closely related to the log-normal distribution.

Here is a simple  interactive example  for a "natural" computation of the mean.

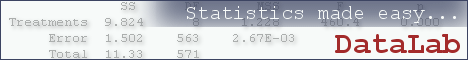
Please note that there are different notations for the mean: the mean of a population is denoted by μ, whereas the mean of the scores of a sample is denoted either by m, or by .

The mean is a good approximation of the central tendency for unimodal symmetric distributions, but can be misleading in skewed or multimodal distributions. Therefore, it can be useful to specify other additional measures of location for skewed distributions (i.e. the median is more robust in case of skewed distributions or or in case of outliers). Another way to deal with outliers is to use a trimmed mean, which is calculated after the lower and upper fraction (typically 5%) of the values have been discarded.

Hint 1:     It can be shown that the sum of squared deviations of sample scores from their mean is lower than the squared deviations from any other value.

Hint 2:    The mean is also often related to the accuracy of an experiment. See the following  interactive example  to get an impression of accuracy versus precision.

1

A popular question to which most people intuitively provide a wrong answer is, for example, the following problem: a car travels the route from Vienna to Salzburg at a constant speed of 120 km/h. On the return trip the car goes at a constant speed of 80 km/h. What is the average speed for the entire journey? If you spontaneously decide in favor of 100 km/h you are wrong (but in good company with most other people) - the average speed is 96 km/h, which is the harmonic mean of 80 and 120 km/h.